2.6 Interval Inversion and Compound Intervals

Now that we have a basic understanding of interval size and quality, let’s learn some skills that will later help us use intervals to construct chord progressions and interpret harmonies. In this section, we will go over interval inversions and compound intervals. 

Interval Inversions

When we talk about intervals, we are always talking about ascending intervals. For example, a C to an E is a Major 3rd. But what about an E to a C? Sure, we could figure out by looking at the notes that an E to a C is a minor 6th. What interval would a descending minor 3rd be? There must be some relation between a descending interval and an ascending interval.

Interval inversion is the process of taking an interval (the distance between two pitches) and flipping it upside down! 

For example, the inversion of a perfect fifth (an interval consisting of seven half steps) is a perfect fourth (an interval consisting of five half steps). The inversion of a major third (an interval consisting of four half steps) is a minor sixth (an interval consisting of eight half steps).

All you have to do to invert an interval is subtract the interval by 9, and then give the inverted interval the opposite quality as the original interval. For example, the inversion of an A5 would be a d4, and the inversion of an M3 would be a m6. The inversions of perfect intervals are also perfect. 

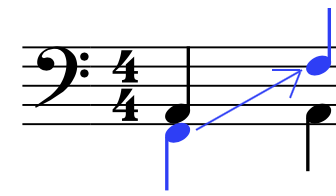
Here's an example of a major third (F-A) being inverted:

Let's look at a few more examples of inversions on a staff:

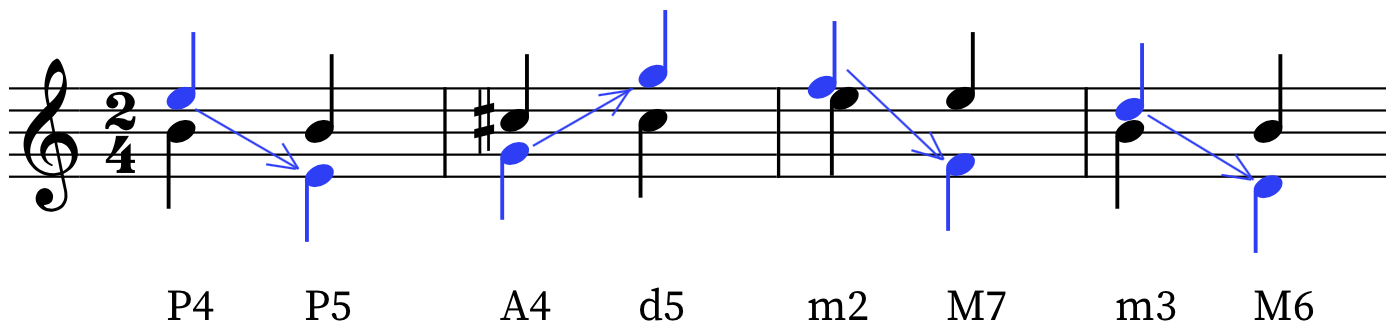
Your turn! Practice inverting these intervals by using the tricks above (answers at the end of the chapter):

• diminished 4th
• major 6th
• minor 7th
• perfect 5th
• major 2nd
• augmented 4th

There are two ways to interpret an interval inversion. First is that if you have two notes that are some interval apart, switching around those two notes gives the interval inversion. A common example that you should remember is the P5 and P4 inversions. If you have a perfect 5th, say G to D, and you switch the notes around, you get a perfect 4th. 

The other way to interpret interval inversions is that they are the descending intervals. Let’s take the same example. We know that the inversion of a perfect 5th is a perfect 4th. If I want to go up by fifths starting at G, I get G, D, A, etc. What if I want to go down by perfect 5ths? Based on the inversion, going down by perfect fifths is the same thing as going up by perfect 4ths. So, I get G, C, F, etc. 

Compound Intervals 

Compound intervals are intervals that span more than one octave. In music, we sometimes have very big intervals, but saying that two notes are a 24th apart is not as meaningful as saying that two notes are 3 octaves and a 3rd apart. The 3rd creates the unique sound of the interval – moving a note up or down an octave doesn’t really matter too much in terms of interpreting consonance, dissonance, and interval quality. 

Here are a few compound intervals:

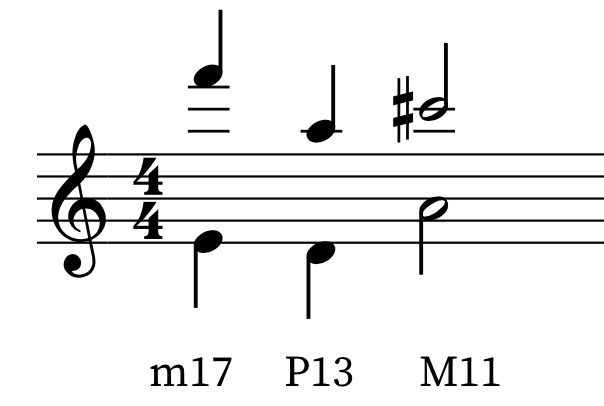
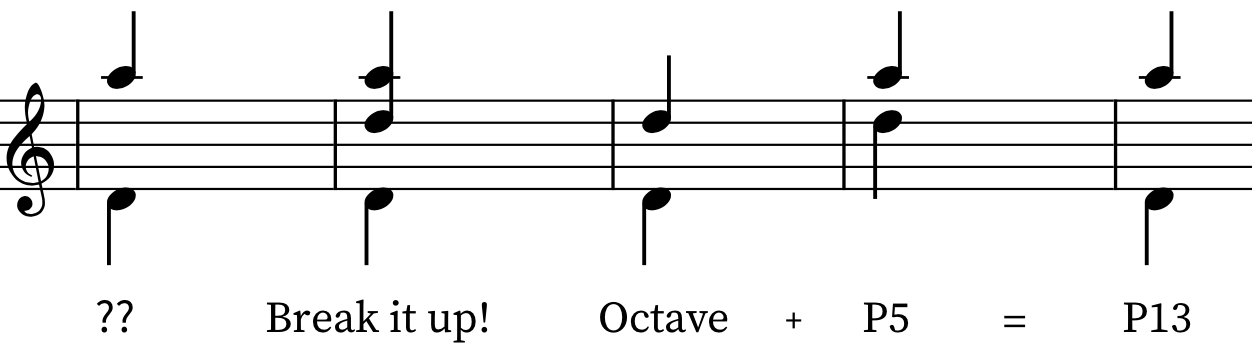
Why is a 24th three octaves and a 3rd and not just 3 octaves? One octave is 8 notes, right? To answer this question, try considering an octave and a 1st. That’s still just an octave, since a P1 is a perfect unison. So, a 9th is actually an octave and a 2nd. 

This happens every time you add an octave. You might want to remember that P1 is a perfect unison, and the octaves are 1 + 7n, where n > 0. An 8th, 15th, 22nd, 29th, etc. are all perfect octaves. From there, you can figure out the compound interval by subtracting your interval from the largest possible octave and adding 1. For example, a 13th is an octave plus a 6th. You might also refer to this as a “compound 6th.” 

The interval quality stays the same when you compound intervals. If you have a minor 13th, it will be a compound minor 6th, and if you have a Major 13th, you have a compound Major 6th. 

🦜 Polly wants a progress tracker: Can you identify the interval that spans from an F# to the B an octave above? What about a G that reaches to the Db two octaves above?

Answers:

• augmented 5th
• minor 3rd
• major 2nd
• perfect 4th
• minor 7th
• diminished 5th